Interstellar Coordination
When it comes to locating objects in the night sky, how does one convey its precise position? The sky is divided into a scattered pattern of 88 constellations of various sizes and shapes covering every square degree of the sky with no gaps in between. No matter where a celestial object is located it will be within the boundaries of some constellation. However, the largest constellations stretch more than a quarter way around the sky so how can one be more specific?
Brighter objects can sometimes be located using terrestrial terms, such as “30 degrees above the southwest horizon 45 minutes after sunset.” But most of the celestial objects of interest to telescope observers are not that bright or may even be invisible to the unaided eye.
The solution astronomers came up with is establishing a standard yet simple coordinate system which can be used for mapping out the sky. In order for this to work, some simplifying assumptions and thought exercises are required to understand and comprehend it.
For the sake of simplicity, the entire sky may be assumed to be projected onto the inside of a hollow sphere (the “celestial sphere”) with the observer located at its center. For most purposes we can ignore the fact that the observer is in reality located some finite distance from the sphere’s center, standing on the surface of a concentric rotating sphere, the Earth.
The only time that the radius of the Earth is of any importance is while determining positions for near-Earth objects such as the moon. Indeed, two Earthbound observers a reasonable distance apart will each see the moon projected against the celestial sphere at different points because of the “parallax effect.” But we’ll ignore that here because we’re interested in pinpointing objects far away from Earth such as the stars.
Also, although space is three-dimensional with some objects being closer to us than others, for simplicity’s sake we can assume that all celestial objects are attached to the sphere’s inside surface whose radius may be thought of as being infinite.
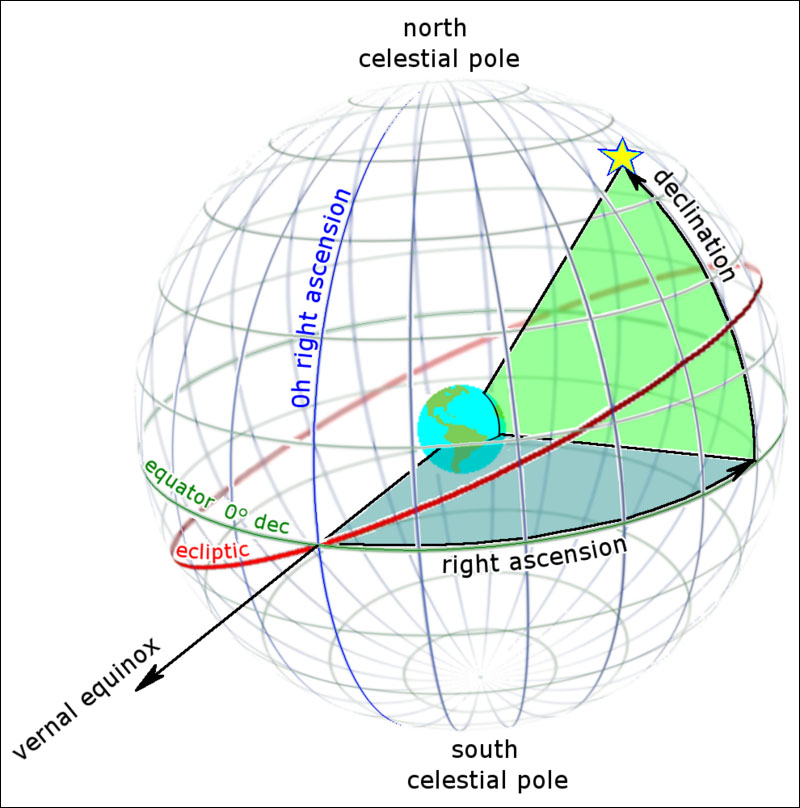
Visualize extending Earth’s rotational axis in an imaginary line to where it intersects the celestial sphere at two points – the north celestial pole (NCP) and south celestial pole (SCP). At our latitude north of the equator we can only see the NCP. The SCP is blocked from our view by the Earth itself. The “celestial equator” is an imaginary ring that runs around the celestial sphere halfway between both poles.
The celestial equator may also be thought of as a plane defined by Earth’s terrestrial equator extending outward to where it intersects the celestial sphere. Mintaka, the rightmost star of the 3-star “belt” in the constellation of Orion happens to be located very near the celestial equator.
“Declination” is the north-south coordinate of an object and is a direct analog to Earth’s own Latitude system. The angular distance of the object north or south of the celestial equator is its declination. If located directly on the celestial equator its declination is 0°. Objects north of the celestial equator have a positive declination; those south of it are negative. The declination of the NCP is +90°; the SCP is -90°.
That’s simple enough. The east-west coordinate is a little bit trickier.
Imagine an orange having 24 equal slices. Next imagine that the orange expands until its completely fills the celestial sphere. Orient the orange so that its pedicel (where it once connected the tree) is at the NCP. The slices now represent 24 hours of “right ascension” (R.A.) where the width of every slice is one hour of R.A., or 15° (360° divided by 24). Each hour in turn is divisible by 60 arcminutes and each arcminute in turn by 60 arcseconds. R.A. is the other coordinate of the system.
The only remaining problem is how to orient the slices. How is the zero hour defined and where does it map to in the sky? The answer is simple but requires another mental simplification.
Earth travels once around the sun each year. From our perspective, the projected position of the sun against the celestial sphere traces a yearly circle around the sky known as the “Ecliptic.” The Ecliptic crosses the celestial equator at two points. One point marks the Vernal (or spring) Equinox and the other the Autumnal (or fall) Equinox. The sun crosses these imaginary points on the first day of spring and the first day of fall, respectively.
This point of the Vernal Equinox on the celestial equator defines the zero hour of R.A. in our coordinate system. R.A. increases eastward and is measured in hours and minutes of R.A.
The celestial location of an object can be specified using only two coordinates, R.A. and declination. For example, the star Betelgeuse representing Orion’s shoulder is located at R.A. 5h 55.2m, Declination +7° 24’.
When an object’s coordinates are known, its position can be plotted on a star map or atlas. Or, if you have a computer-controlled telescope, just enter in the coordinates into the keypad and go.
R.A. and declination is but one systems for celestial coordinates. There are others, such as (1) Ecliptic coordinates in which the Ecliptic plane is the celestial equator; (2) galactic coordinates where the celestial equator is defined by the plane of our Milky Way galaxy; and of course (3) simple X,Y,Z coordinates. Each system has its own special uses.
In local news the Bear Branch Nature Center (BBNC) and its planetarium remain closed due to an ongoing pandemic. However, the Westminster Astronomical Society (WASI) has been hosting monthly virtual planetarium shows using Zoom. For more information, go to westminsterastro.org and look under “Next Month’s Presentation” for planetarium show times and Zoom connectivity information.
–Curt Roelle
